STATES OF MATTER

STATES OF MATTER

The  three important states of the matter are (i) Solid state (ii) Liquid state (iii) Gaseous state, which can exist together at  a particular temperature and pressure e.g. water has three states in equilibrium at 4.58 mm and 0.0098ºC.

PLASMA STATE : It is the gaseous mixture of electrons and positive ions existing at extremely high temperatures (in the interior of stars) or internal electrical fields in discharge tubes.

SINGLE SUPER ATOM STATE : At extremely low temperature, all atoms lose their identity and get condensed into a single entity behaving like a single super atom.

MEASURABLE PROPERTIES OF GASES

Four measurable properties are:

1. Mass :  It is expressed in grams or kg. 1 kg=103 g moles of

gas =

2. Volume : It is equal to the volume of the container and is expressed in terms of litres (L), millilitres (ml), cubic centimeters , cubic meters (m3) or cubic decimeters (dm3).  

3. Pressure : It is equal to force per unit area and expressed in the units such as atmosphere, millimetres (mm), centimetres (cm), torr, bar etc. SI unit of pressure is pascal (Pa) or kilopascal (kPa)

4. Temperature : It is measured in celsius scale (°C)or in Kelvin scale (K). SI unit of temperature is Kelvin (K) or absolute degree T (K) =

GAS LAWS

BOYLE’S LAW

The volume of a given mass of a gas is inversely proportional to its pressure at constant temperature.

, a constant

Value of k depends on mass, temperature and nature of gas.

When mass and temperature are the same we have

ISOTHERMS  

Graphs of V vs P or PV vs P at constant temperature are known as Isotherm.

Graphical representation of Boyle's law

CHARLE’S LAW

The volume of the given mass of a gas increases or decreases by of its volume at 0°C for each degree rise or fall of temperature at constant pressure.

or

The volume of a given mass of a gas is directly proportional to the absolute temperature at constant pressure.

ABSOLUTE ZERO

If in the above equation we put the value of t as –273.15 ºC the volume of the gas will be zero. It means gas will not exist, which is not possible. In fact all gases get liquified before this temperature is reached. This hypothetical or imaginary temperature at which the gases occupy zero volume is called absolute zero.

ISOBARS

A graph of V vs T at constant pressure is known as Isobar.

GRAPHICAL REPRESENTATION OF CHARLE’S LAW

GAY LUSSAC’S LAW : (AMANTON’S LAW)

The pressure of a given mass, of gas increases or decreases by   of its pressure at 0°C for each degree rise or fall of temperature at constant volume.

or

The pressure of a given mass of a gas at constant volume is directly proportional to absolute temperature

ISOCHORES

A graph of P vs T at constant volume is known as Isochore

GRAPHICAL REPRESENTATION OF GAY LUSSAC’S LAW

AVOGADRO’S LAW

The volume of a gas is directly proportional to number of molecules or moles at constant temperature and pressure.

  at constant T and P

GRAPHICAL REPRESENTATION OF AVOGADRO’S LAW

IDEAL GAS EQUATION

.

This is known as ideal gas equation. R is known as universal gas constant.

IDEAL GAS

The gas which obeys the equation  at every temperature and pressure range strictly.

REAL OR NON-IDEAL GASES

Since none of the gases present in universe strictly obey the equation , hence they are known as real or non ideal gases. Real gases behave ideally at low P and high T.

DENSITY OF A GAS

We have ,

Further,

The above equation shows that density of a gas depends on P and T.

Unit of density of gas :

It is usually expressed in gm/ litre

VAPOUR DENSITY (V.D.)

It is the ratio under similar conditions of P and T

Unit of vapour density : It is a ratio and has no unit.

NATURE OF GAS CONSTANT, (R)

   

    = Work done per degree per mole

Deviation from ideal behaviour in terms of compressibility factor Z

Deviation from ideal behaviour in terms of PV-P curve

NUMERICAL VALUE OF R

As the work can be expressed in different systems of units, R will have different values.

1. In litre - atmosphere :

At NTP,  P = 1atm, V=22.4 litre and T= 273.15K

2. In cgs system :

P = 1 atm = 1×76×13.6×980 dyne cm-2; V = 22400 cm3;
T = 273.15 K

3. In mks or SI units :  

Unit of R is JouleK–1

107 ergs = 1 Joule or R = 8.314 Joule K–1    

NTP OR STP AND SATP

Normal or standard temperature & pressure means 0°C or 273.15 K and 1 atm pressure.

Normal boiling : At 1 atm pressure the boiling temperature is called Normal boiling point.

Standard boiling point : At 1 bar pressure the boiling temperature is called standard boiling point.

Normal boiling point of water is 100 °C (373.13 K)

Standard boiling point of water is 99.6 °C (372.6K)

Standard temperature and pressure (STP)

273.15 K(0 °C) temperature

1 bar (105 pascal) pressure

Molar volume at STP =  22.71098 L mol–1

Normal temperature and pressure (NTP)

273.15 K(0 °C) temperature

1 atom (1.01325 pascal) pressure

Molar volume at NTP =  22.413996 L mol–1

Standard ambient temperature and pressure (SATP)

298.15 K (25 °C) temperature

1 bar (105 pascal) pressure

Molar volume at SATP  = 22.784 L mol–1

BOLTZMANN CONSTANT (k)

It is the gas constant  per molecule thus ; Where R=gas constant., N0= Avogadro number.

Value of  

DALTON’S LAW OF PARTIAL PRESSURE

At constant temperature the total pressure, exerted by a mixture of non reacting gases, is the sum of partial pressure of each gas

Partial pressure p of a gas = mole fraction of the gas ×  total pressure.

For a gas A,   pA = xA ×  P

If  n1,n2,and n3 are moles of non reacting gases filled in a vessel of volume V at temperature T.

The total pressure P is given by  

This is the equation of state of a gaseous mixture.

RELATIONSHIP BETWEEN TOTAL PRESSURE AND INDIVIDUAL PRESSURE

On mixing of gases at constant temperature. If a gas A at pressure P1 and volume V1 is mixed with gas B at pressure p2 and volume V2 at same temperature, then

Total pressure,

AQUEOUS TENSION

It is the pressure exerted by water vapour at a particular temperature. It depends upon temperature.

PRESSURE OF A DRY GAS

When a gas is collected over water,  its observed pressure is equal to the sum of the pressure of dry gas and the pressure of water vapour (aqueous tension), then

Pressure of dry gas = pressure of moist gas  – aqueous tension

DIFFUSION

The tendency of every gaseous substance to distribute itself uniformly throughout the available space is known as diffusion. It also takes place through a porous vessel.

EFFUSION

The movement of a gas through a small hole when it is subjected to pressure is known as effusion.

GRAHAM’S LAW OF DIFFUSION  (OR EFFUSION)

At constant temperature and pressure, the rate of diffusion or effusion of a gas is inversely proportional to the square root of its density.

Rate of diffusion ∝

If and   are rates of diffusion of two gases and and their respective densities then,

Rate of diffusion

If volume diffused is same

If time of diffusion is same  

Effect of pressure on the rate of diffusion.

Rate of diffusion ∝ pressure, therefore,

APPLICATIONS OF GRAHAM’S LAW OF DIFFUSION

1. Determination of densities and molecular masses of unknown gases.
2. Separating the gases having different densities.
3. Separation of Isotopes

ATMOLYSIS

The phenomenon of separation of a mixture of gases due to difference in their rates of diffusion is called atmolysis.

KINETIC THEORY OF GASES

Postulates of kinetic theory of gases.

1. A gas consists of large number of tiny particles called molecules.
2. Volume occupied by gas molecules, is negligible as compared to the total volume of gas.
3. There is continuous rapid random motion of gas molecules. The molecules collide with each other and against walls of container.
4. The molecules are perfectly elastic bodies and there is no loss of kinetic energy during collisions.
5. There are no attractive forces between the molecules of gas.
6. The pressure exerted by a gas is due to bombardment of gas molecules against the walls of the container.
7. The different molecules possess different velocities and hence different energies. The average K.E. is directly proportional to absolute temperature.

KINETIC GAS EQUATION

Based upon the postulates of Kinetic theory of gases, the kinetic gas equation is

where m = mass of a gas molecule, N= number of molecules,
U= Root mean square velocity.

KINETIC ENERGY OF GAS

It can be obtained from kinetic gas equation

KE of one molecule

=

of a gas

• KE of n moles of gas = 3/2 nRT
• At absolute zero, KE is zero

MAXWELL’S GENERALISATION

Kinetic Energy of translation of ideal gas is directly proportional to absolute temperature of gas or its pressure and is independent of the nature of gas.

THERMAL MOTION

The molecular velocity of a gas is proportional to square root of the absolute temperature. The molecular motion is called thermal motion of molecules.

CALCULATION OF MOLECULAR VELOCITIES OF GASES

1. Calculation of velocity when temperature alone is given :

From Kinetic gas equation,

; 

2. Calculation of velocity at NTP

It is given by the formula,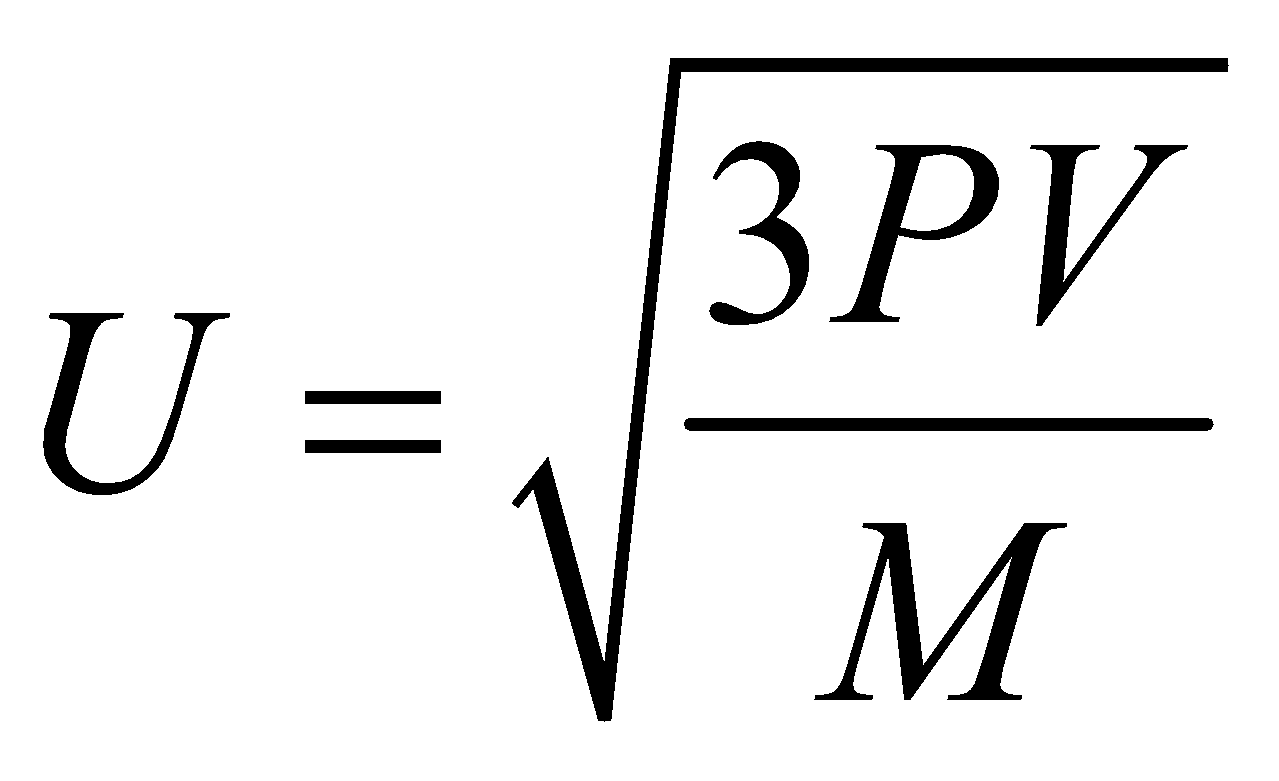      

AT  NTP, P=dynes cm-2,  (molar volume)

3. Calculation of velocity when pressure and density are given.

It is given by the following formula,

=         ()

4. Calulation of velocity when  pressure and temperature are given but are not the same as NTP

;  In such case molecular volume (22400ml) is converted into the volume under given conditions of T and P using relation,

MAXWELL’S DISTRIBUTION OF VELOCITIES

The molecules present in a given sample of gas move with different velocities in all possible directions. Velocities and directions of molecules keep on changing due to intermolecular collisions. Hence  it is impossible to find out the individual velocity of each molecule. It is however possible to predict fraction ( ) of the total  number of molecules having specific velocities at a particular  temperature. As shown by the curve,

The gases show ideal behavior at low presence/large volume. Since the volume of molecules can be neglected and at high temperature since intermolecular forces decrease.

Fraction of molecules having very small or very high velocities is very low. No molecule has zero velocity. Fraction of molecules  possessing a particular velocity at a particular temperature is constant. The different velocities possessed by gas molecules are:

1. Most probable velocity () : It is the velocity possessed by maximum fraction of gas molecules at a particular temperature

Mathematically,

2. Average velocity () : This is the average of the different velocities of all the molecules.

where c1,c2,c3 etc. are  individual velocities of n1,n2,n3 molecules.

Mathematically, =

3. Root mean square velocity (U) : It is the square root of the mean of the square of the different velocities of the molecules

;                  

Mathematically, U =

RELATIONSHIP BETWEEN DIFFERENT TYPES OF VELOCITIES :

 =

         ==

Calculation of root mean square velocity (Urms) :   As already discussed, the Urms can be calculated by any of the following relations:

Urms =

Ratio of Urms for two  different gases at the same temperature

Ratio of Urms for the same gas at different temperature

EFFECT OF TEMPERATURE ON DISTRIBUTION OF MOLECULAR VELOCITIES

Most probable velocity increases with the rise in temperature but fraction of molecules possessing such velocity decreases.

Deviation from ideal behaviour of gas :

At  high pressure and low temperature the gases deviate considerably from the ideal behaviour. Deviation can be expressed in terms of compressibility factor (Z), expressed as

In case of ideal gas,

In case of real gas,

Negative deviation

In such case, Z < 1, gas is more compressible.

Positive deviation

In such case, Z >1, gas is less compressible.

Factors affecting the deviation :

The factors affecting the deviation are:  

1. Nature of the gas :  In general, the most easily liquefiable and highly soluble gases show larger deviation.
2. Pressure : The deviation is more at high pressure. CO2 and N2 show negative deviation at low pressure and positive deviation at high pressure.
3. Temperature : The deviation is more at low temperature. H2 and He always show positive deviations at 0°C

BOYLE’S TEMPERATURE

Temperature at which a real gas exhibits ideal  behaviour for considerable range of pressure is called Boyle’s temperature. Mathematically,

,   where a and b are Van der Waal’s constants

1. Boyle temperature is different for different gases
2. Below Boyle’s temperature a gas shows negative deviation.
3. Above Boyle’s temperature a gas shows positive deviation

CAUSE OF DEVIATION FROM THE IDEAL BEHAVIOUR

It is due to two faulty assumptions of Kinetic theory of gases particularly not valid at high pressure and low temperature.

1. Volume occupied by the gas molecules is negligible as compared to the total volume of gas.
2. There are no attractive forces between the gas molecules.

VAN DER WAAL'S EQUATION

After volume & pressure correction, van der Waals obtained the following equation for n moles of a gas

, For one mole

Excluded volume or co-volume, (b)

The constant b in van der Waal's equation is known as excluded volume or co-volume. It is nearly four times the actual volume occupied by the gas molecules.

Excluded or co-volume (b) = 4 × actual volume of gas molecules.

Magnitude of attractive forces  between gas molecules (a)

It is given by van der Waals constant ‘a’. Different gases have different value for ‘a’.

(i) The greater the value of ‘a’, the greater the strength of van der Waals forces.

(ii) The greater the value of ‘a’, the greater is the ease with which a gas can be liquefied.

Units for Van Der Waal's constant:

Pressure correction,

Volume correction,

DISCUSSION OF VAN DER WAAL’S EQUATION

Van der Waal’s equation for one mole of gas

1. At low pressure and ordinary temperature : V is very large and b can be neglected  then

or ;

Therefore Z is less than 1.

Hence at low pressure the gases show negative deviation.

2. At high pressure and ordinary temperature : At high pressure, volume V will be quite small. The quantity  become  negligible but ‘b’ cannot be ignored.

or ; Therefore Z is more than 1.

At high pressure the gases show positive deviation

Note : At some intermediate range of pressure and ordinary temperature the gas shows the ideal behaviour.

3. At low pressure and high temperature : The effect of   and b is negligible. We have or

Therefore Z = 1. Hence gas shows ideal behaviour.

4. Exceptional behaviour of Hydrogen and Helium :
   Due to their small size, the attractive forces between the molecules are too small, is negligible, then
1. 
   

Hence they always show positive deviation.

LIMITATION OF VAN DER WAAL’S EQUATION

There is specific range of temperature and pressure, to apply the equation. It deviates at too high pressure and too low temperature.

Other equations of states :

1. Dieterici equation :
2. Berthelot’s equation : 
3. Clausius equation :

Where c is a new constant

4. Kammerling onne's equation (virial equation):

1. The coefficients A,B,C,D, etc are known as first, second, third, fourth virial  coefficients
2. At low pressure only A (which  is equal to RT) is important, the others cancel out.
3. A is always positive and increases with rise of temperature.
4. At Boyle temperature B=0
5. Relation between B and van der Waals constant is  

CRITICAL PHENOMENON AND LIQUEFACTION OF GASES

Increase of pressure and decrease of temperature tend to cause liquefaction of gases. The effect of temperature is, however more important.

CRITICAL TEMPERATURE (TC)

It may be defined as the temperature above which no gas can be liquefied howsoever  high the pressure may be, critical temperature of CO2 is 31.1°C.

Critical temperature (Tc)  of some gases,

He 5.2 CO2 304.1 CH4 190.2

H2 33.2 N2O 309.6 HCl 324.5

N2 126.0 NH3 405.5 H2S 373.5

CO 134.4 Cl2 417.1 Ar 150.7

O2 154.3 SO2 430.3

CRITICAL PRESSURE (PC)

At critical temperature the pressure needed to liquefy a gas is known as critical pressure.

CRITICAL VOLUME (VC)

The volume occupied by one mole of a gas at critical temperature and critical pressure is known as critical volume.

Relationship between critical constants and van der Waal’s constant:

Relationship between boiling point and critical temperature:

Boiling point =critical temperature.

Compressibility for 1 mole of gas at critical state:

,   almost constant.

JOULE THOMSON EFFECT

When a gas under high pressure is allowed to expand adiabatically into a region of extremely low pressure, it suffers change of temperature. The phenomenon is known as Joule -Thomson effect.

CONDITION FOR JOULE-THOMSON EFFECT (INVERSION TEMPERATURE)

In Joule -Thomson effect, cooling is observed only if the gas is present below certain temperature known as inversion temperature, Ti. It is characteristic of each gas and related to Van der Waal’s constant a and b  as,

At exact inversion temperature there is no Joule-Thomson effect. Above inversion temperature, there is heating during Joule-Thomson effect. Below inversion temperature there is cooling during Joule-Thomson effect.

Inversion temperature for hydrogen = –80°C and for He = –240°C. Joule-Thomson effect is zero in an ideal gas and enthalpy remains constant. When an ideal gas expands in vacuum, it does no work  i.e. W=0 ; (Adiabatic condition). Hence internal energy of a given quantity of an ideal gas at constant temperature is independent of its volume.

(for an ideal gas) ;  This quantity is called internal pressure and is positive for real gases.

Thermodynamically an ideal gas may be defined by following the equations.

1. PV = constant, at constant temperature.
2. 

JOULE-THOMSON COEFFICIENT () :

The temperature change produced per atmosphere drop in pressure under constant enthalpy conditions on passing a gas through the porous plug is called Joule-Thomson coefficient

The quantity    is called Joule -Thomson coefficient.

1. Joule Thomson Coefficient   is positive for real gases, (cooling)

Or Joule Thomson Coefficient will be positive when

2. When Joule Thomson Coefficient    is negative, (heating)

Or Joule Thomson Coefficient will be negative when

3. When Joule Thomson Coefficient is zero, (no heating or cooling)

Or Joule Thomson Coefficient will be zero when

Since a, b and R are constants, the sign of Joule-Thomson Coefficient will depend only upon the temperature at which the gas is being allowed to expand. The temperature at which the Joule-Thomson Coefficient changes sign is known as the inversion temperature.

LAW OF CORRESPONDING STATES

When the values of pressure, volume and temperature are expressed as fractions of the corresponding critical values we have.

where , and are termed, the reduced  pressure, the reduced volume and the reduced temperature respectively. If we replace P,V and T by Pc, Vc and Tc in van der Waal’s equation and put the values of Pc, Vc and Tc terms of a, b and R we get,

If the two substances have the same reduced temperature and the same reduced pressure, they will have the same reduced volume. The statement is known as the law of corresponding states.

HEAT CAPACITY OF SYSTEM

For gases it can be at constant volume or at constant pressure.

1. Heat capacity at constant volume (CV) is defined as  the increase in internal energy of a gas per degree rise of temperature.

2. Heat capacity at constant pressure (Cp) is defined as increase in enthalpy of a gas per degree rise of temperature.

For one mole of gas, the heat capacities at constant volume and constant pressure are denoted by Cv and Cp and are termed as molar heat capacities.

RELATION BETWEEN CP AND CV

Cp– Cv = R

R = 1.987 cal or 8.314 Joule

R= PΔV = Work done by one mole of an ideal gas in expansion at constant pressure when heated through 1°C.

MOLAR HEAT CAPACITIES FOR POLYATOMIC GASES

The values   and are for monoatomic gases like He, Ar etc. where the energy supplied increases translational kinetic energy only. In polyatomic gases heat supplied is utilised to increase vibrational and rotational energy also. Thus we have

For monoatomic gas, x = 0,  

for diatomic gases, x = R,

for triatomic gases, ,

COLLISION PROPERTIES

1. Molecular diameter or collision diameter :  The distance between the centers of the molecules at the point of their closest approach.

2. Collision frequency (Z) : The number of collisions experienced by molecules per cc of a gas per second is known as collision frequency  of gas,

= average velocity

N = number of molecules per cm3

= collision diameter

At constant pressure,

At constant temperature,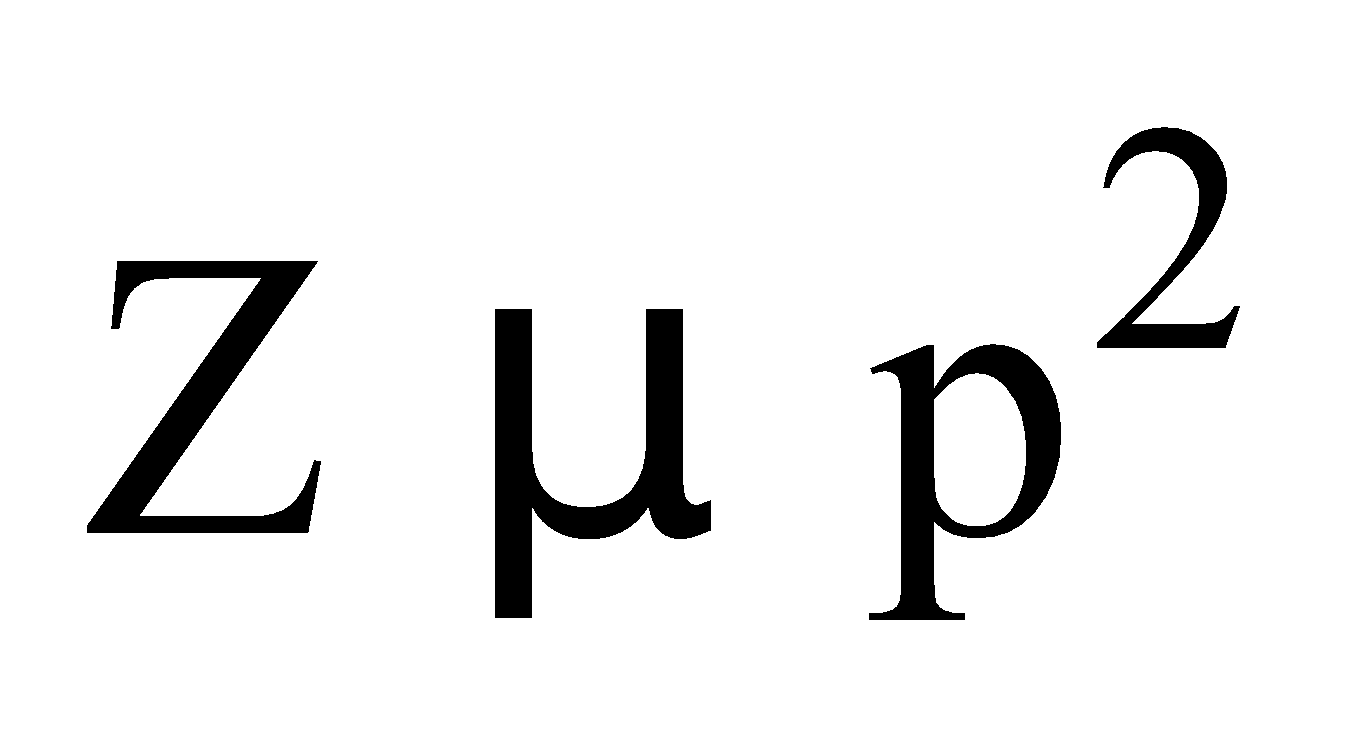  

3. Collision number (Z1) :  Number of collisions undergone by a molecule with other molecules per second present in 1cm3.

Z1 = Collision number =

= diameter of molecules,  = Average velocity,
N = Number of molecules per unit volume of the gas

4. Mean free path (l) : The average distance travelled by the molecule between two successive collisions

At constant pressure,    

At constant temperature, l

VOLUME COEFFICIENT

It is defined as the ratio of the increase in volume of  the gas at constant pressure per degree rise of temperature to its volume at 0°C

V0 =Volume of a given mass of a gas at 0°C

Vt= Volume of a given mass of a gas at t°C

The value of v is found  be 1/273 for all gases (charle's law)

Therefore,

PRESSURE COEFFICIENT ()

It is defined as the ratio of the increase in pressure of the gas at constant volume per degree rise of temperature to its pressure at 0°C

P0=Pressure of given mass of gas at 0°C

Pt=Pressure of given mass of gas at

The value found by Ragnault & Gay Lussac was in the vicinity of   for all gases and hence

AMAGAT LAW OF PARTIAL VOLUME

The total volume of a mixture of non reacting gases at constant temperature and pressure is equal to the sum of the individual partial volumes of the constituents

V(total)= V1+V2+V3+.....+Vn  

LOSCHMIDT NUMBER

It is the number of molecules present in 1cc of a gas or vapour at STP. Its value is  2.617×1019 per cc.

AVERAGE MOLECULAR WEIGHT OF A GASEOUS MIXTURE

Mmix=

ni= number of moles

Mi= molecular weight of each component

BAROMETRIC FORMULA

The decrease in the atmospheric pressure with increase in altitude given by the following expression is called barometric formula.

ln

P0=Pressure at the sea level

P = Pressure at height h

= Average molecular mass of air,

g = acceleration due to gravity, 9.8 ms-2

R = gas constant ( 8.314 JK-1 mol-1)

T= Temperature in kelvin

ESCAPE VELOCITY

Velocity required by an object to escape from the gravitational field of a body. It is given by

AVERAGE KINETIC ENERGY

It is KE of a single molecule, and , where k= Boltzmann constant

Total KE =N (average KE), N ()  =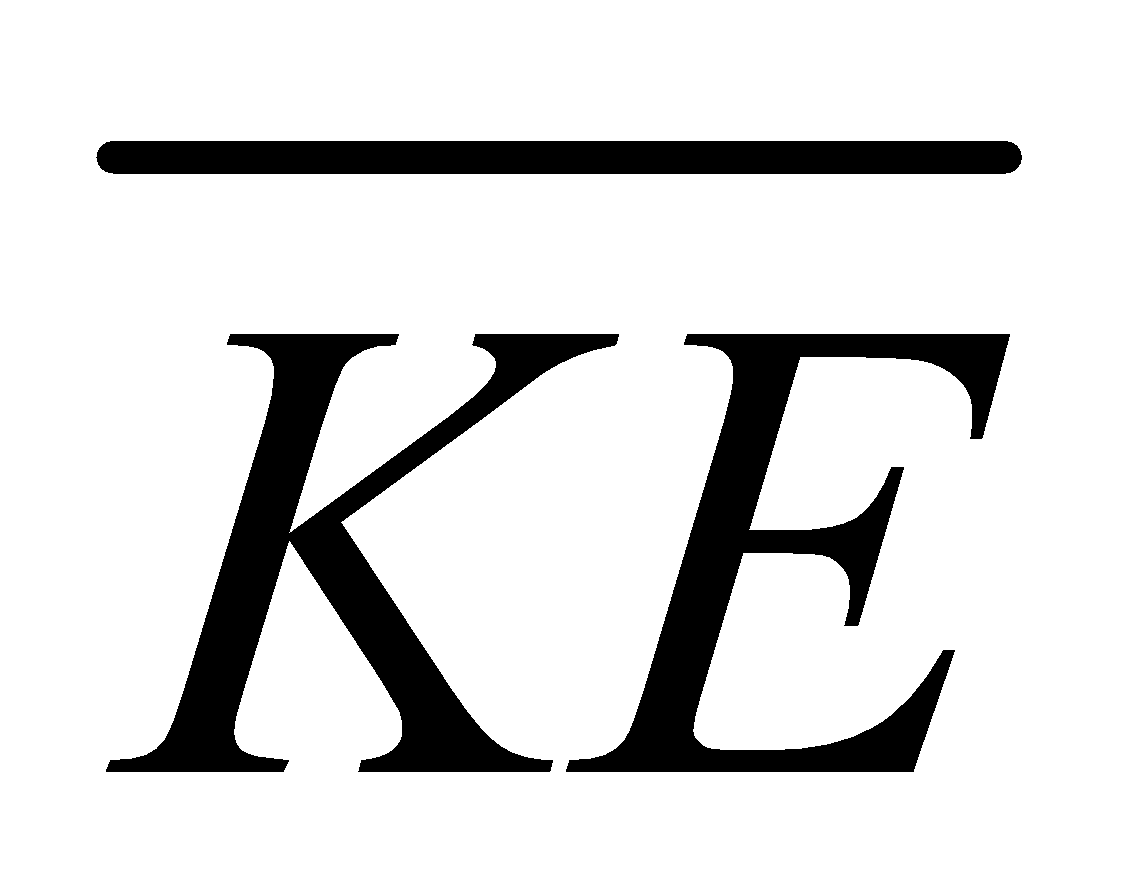

LIQUEFACTION OF GASES

Liquefaction of gas can be achieved by

1. Increasing pressure : It increases attraction among molecules.
2. Decreasing temperature : It decrease Kinetic energy of molecules. The temperature of gas must be lower than its critical temperature, TC.
   

Liquefaction is further based on the following principles

• Cooling by freezing mixture : eg NaCl & ice (-22°C), CaCl2 & ice(-55°C), KOH & ice (-65°C)
• Cooling by adiabatic expansion (Claude’s Method) : The gas in this process suffers a loss in temperature. Work is done by the gas at the cost of internal energy and temperature is lowered.
• Cooling by Joule-Thomson effect ( Linde’s method) : Expansion of a gas through a small jet under adiabatic conditions results in cooling and liquefaction of gas

LIQUID STATE

1. Liquid state of the matter is the intermediate state between the gaseous and the solid state.
2. In liquids the molecules of the matter are held together by strong intermolecular forces in comparison to those in gases.
3. On the basis of kinetic molecular model, the liquid state is described as follow:
1. A liquid is composed of small molecules.
2. The molecules of the liquid are held closer by some kind of intermolecular forces.
3. The intermolecular forces are not very strong and thus the molecules are always in constant random motion.
4. The average kinetic energy of molecules of a liquid is directly proportional to their absolute temperature.
4. Properties of the liquid :

Most of the physical properties of liquids are controlled by the strength of intermolecular attractive forces existing between molecules of a liquid. These intermolecular forces arranged in order of their increasing strength are

London forces/induced dipole<dipole attraction< Hydrogen bonding

1. Shape: Liquids have no definite shape of their own. It acquires the shape of the container in which  it is put.
2. Volume: Liquids have a definite volume.
3. Density: The densities of liquids are nearly 1000 times more than the densities of the gases under  identical conditions.
4. Compressibility: Liquids are very less compressible compared to gases.
5. Diffusion: Liquids diffuse like gases but the process of diffusion (also known as intermixing) is much slower.
6. Evaporation: The process of conversion of a liquid into its vapours (i.e.) gaseous state) at room temperature is known as evaporation.

VAPOUR PRESSURE

At equilibrium state the pressure exerted by vapour phase is called vapour pressure at a specific temperature.

• The magnitude of vapour pressure depends upon the following two factors.
1. The liquids having weak intermolecular attraction have greater tendency of escaping from the liquid surface in comparison to liquids having stronger forces of intermolecular attraction. For example, the vapour pressure of ether and acetone is more than that of water or acetic acid at any specific temperature.
2. The vapour pressure of a liquid increases with the increase in temperature.
• The vapour pressures of a given liquid at two different temperatures may be compared using Clausius Clapeyron equation

where P1and P2 are the vapour pressures at temperature T1 and T2 ΔH is the heat of vapourisation and R is molar gas constant.

• The temperature at which the vapour pressure of liquid becomes equal to atmospheric pressure (or the external pressure) is termed as the boiling point of liquid. For example at 1 atmospheric pressure acetone boils at 56°C. benzene at 80°C, ethyl alcohol at 78.4°C and water at 100°C.
• The temperature at which the solid state and the liquid form of a substance are in equilibrium at one atmospheric  pressure is known as freezing point

SURFACE TENSION

• The surface tension is defined as the force per cm acting perpendicular to the tangential line on the surface of the liquid which tend to compress the surface area.
• The units of surface tension are force per unit length i.e. dynes cm–1 or Nm–1 (in SI units)
• Stronger the forces of intermolecular attraction (cohesive forces) greater is the surface tension.
• The surface tension decreases with rise in temperature or surface tension in inversely proportional to temperature.Surface tension 1/Temperature
• Measurement of surface tension of a liquid by the drop number method is the most convenient method.

VISCOSITY

• Viscosity may be defined as the force of friction between two layers of a liquid moving past one another with different velocities.
• The viscosities of liquids are compared in terms of coefficient of viscosity which is defined as the force per unit area needed to maintain a unit difference in velocities between two consecutive parallel layers which are one cm. apart.
• The units of viscosity are poise (P) where 1P = g cm–1  sec–1. In SI units of viscosity 1P = 0.1 N  sec m–2.
• The liquid having stronger forces of attraction has a higher viscosity.
• With the rise in temperature, viscosity of a liquid decreases because the intermolecular attractive force between consecutive layers decrease as temperature increases.

Tags:

states of matter class 11 states of matter class 9 states of matter class 11 notes states of matter ncert states of matter in chemistry states of matter notes states of matter gases and liquids states of matter neet questions states of matter are states of matter and properties of matter ppt states of matter allen module states of matter are interchangeable states of matter activity states of matter all states of matter are there states of matter allen module neet states of matter basics states of matter byju's states of matter background states of matter book states of matter bose einstein states of matter by david goodstein states of matter by physics wallah states of matter based on kinetic theory states of matter class 11 ncert pdf states of matter class 5 states of matter class 6 states of matter class 9 pdf states of matter class 9 notes states of matter definition states of matter diagram states of matter drawing states of matter difference states of matter definition chemistry states of matter david goodstein states of matter define states of matter depends on states of matter experiments states of matter examples states of matter explanation states of matter exercise states of matter explain states of matter exemplar states of matter eamcet states of matter essay states of matter for kids states of matter formulas states of matter flow chart states of matter for class 5 states of matter for class 3 states of matter formulas pdf states of matter for class 9 states of matter for class 6 states of matter gases and liquids class 11 states of matter grade 4 states of matter gas states of matter grade 6 states of matter grade 5 states of matter gif states of matter game states of matter handwritten notes states of matter hindi states of matter hindi meaning states of matter hands-on activities states of matter how many states of matter has only vibrational motion states of matter handwritten notes for neet states of matter hots questions states of matter images states of matter in hindi states of matter is physical or inorganic states of matter in chemistry class 11 states of matter important topics states of matter in physics states of matter interconversion states of matter jee notes states of matter jee states of matter jee questions states of matter jee notes pdf states of matter jee advanced questions states of matter jee main notes states of matter jee pyq states of matter jee advanced notes states of matter ka hindi states of matter khan academy states of matter kids states of matter kinetic energy states of matter keywords states of matter kcet questions states of matter ks3 worksheet pdf states of matter exam questions states of matter liquid states of matter lesson plan states of matter lesson plan pdf states of matter lesson plan grade 9 states of matter laws states of matter lesson plans 6th grade states of matter liquid definition states of matter lesson plan grade 2 all states of matter all states of matter occupies space all states of matter occupies space and have volume all states of matter occupies space and volume. * all states of matter in chemistry all states of matter with examples all states of matter discovered all states of matter wikipedia states of matter mcq states of matter meaning in hindi states of matter meaning states of matter model states of matter mcq pdf states of matter mind map states of matter mcq for neet states of matter mcqs with answers states of matter ncert solutions states of matter neet notes states of matter notes class 9 states of matter notes pdf states of matter neet questions pdf download states of matter numericals states of matter one shot states of matter online activity states of matter objective questions states of matter online test states of matter other name states of matter objective questions pdf states of matter of fire states of matter of water states of matter pdf states of matter ppt states of matter plasma states of matter project states of matter previous year neet questions states of matter pdf notes states of matter pictures states of matter physics wallah states of matter questions states of matter questions and answers states of matter question bank states of matter questions and answers pdf states of matter quiz states of matter question bank pdf states of matter questions and answers class 5 states of matter quiz grade 5 states of matter revision notes states of matter real life examples states of matter revision states of matter reading comprehension pdf states of matter riddles states of matter review worksheet states of matter review states of matter rap states of matter solid states of matter solid liquid gas states of matter slideshare states of matter simulation states of matter solutions states of matter short notes states of matter song states of matter study adda states of matter triangle states of matter table states of matter types states of matter topics states of matter test states of matter that coexist during melting states of matter toppr states of matter textbook states of matter unacademy states of matter upsc states of matter unacademy jee states of matter unit states of matter unit plan states of matter unit test states of matter unit test quizlet states of matter video states of matter virtual lab states of matter vedantu states of matter video middle school states of matter videos for grade 4 states of matter vocabulary states of matter venn diagram states of matter volume states of matter worksheet states of matter weightage in neet states of matter wikipedia states of matter worksheet grade 7 pdf states of matter worksheet grade 5 pdf states of matter with examples states of matter worksheet pdf states of matter weightage in jee states of matter youtube states of matter year 4 states of matter year 7 states of matter year 5 states of matter year 7 worksheet states of matter year 3 states of matter year 9 states of matter year 8 states of matter 11 states of matter 11th chemistry states of matter 11 notes states of matter 11 ncert solutions states of matter 1st grade states of matter 12 states of matter 15 states of matter 2020 jee mains questions states of matter 2020 neet questions states of matter 22 states of matter 2nd grade states of matter 2nd grade worksheet states of matter 2021 states of matter 2nd grade video states of matter 2nd grade lesson plans states of matter 3d model states of matter 3rd grade states of matter 3d project states of matter 3rd grade lesson states of matter 3rd grade worksheets 3 states of matter examples 3 states of matter for kids 3 states of matter diagram 3 states of matter definition 3 states of matter differences 3 states of matter images 3 states of matter ppt 3 states of matter chart states of matter 4th grade states of matter 4th grade worksheet 4 states of matter plasma 4 states of matter song 4 states of matter examples 4 states of matter in order of increasing energy 4 states of matter diagram 4 states of matter in chemistry 4 states of matter plasma examples 4 states of matter chart states of matter 5th grade states of matter 5e lesson plan 5 states of matter class 9 5 states of matter name 5 states of matter ppt 5 states of matter in chemistry 5 states of matter in hindi 5 states of matter diagram 5 states of matter class 11 5 states of matter in order states of matter 6th grade states of matter 6 mark question 6 states of matter in chemistry 6 states of matter changes 7 states of matter pdf 7 states of matter in chemistry 7 states of matter with examples 7 states of matter and all details about them states of matter 8th class states of matter 8th grade states of matter 9th class states of matter 9th class mcqs